Finite Element Limit Analysis

Key Researchers: Andrei Lyamin

In geotechnical engineering, stability analysis is used to predict the maximum load that can be supported by a geostructure without inducing failure.  This ultimate load, which is also known as the limit or collapse load, can be used to determine the allowable design load by dividing it by a predetermined factor of safety.  The precise value of this factor depends on the type of problem with, for example, lower values being appropriate for slopes and higher values being adopted for foundations. Rather than imposing a factor of safety on the ultimate load to obtain the allowable design load, it is also possible to apply a factor of safety to the strength parameters prior to performing the stability analysis. In some procedures, for example finite element strength reduction analysis, the actual safety factor on the strength can be found for a given set of applied loads and material parameters (which typically comprise the cohesion and friction angle).

A highly effective method for the solution of stability problems in geomechanics is known as finite element limit analysis (FELA), which has been pioneered at the Newcastle Geotechnical Research Group (Sloan 2013). Based on finite elements, the limit theorems of classical plasticity theory and large scale optimisation, FELA permits the exact failure load to be bracketed from above and below, thus providing an in-built error indicator. The lower bound formulation of FELA computes a statically admissible stress field which satisfies equilibrium, the yield criterion, and the stress boundary conditions. Its upper bound counterpart, on the other hand, determines a kinematically admissible velocity field which satisfies the plastic flow rule and velocity boundary conditions. Both the lower bound and upper bound formulations lead to large-scale nonlinear optimisation problems which are solved very efficiently using bespoke interior-point algorithms.

The latest version of the FELA software can handle problems with inhomogeneous soil profiles, anisotropic strength characteristics, complex loading and boundary conditions, soil-structure interaction and three-dimensional geometries. Moreover, it incorporates robust mesh adaptivity schemes, the effects of steady-state pore water pressures, and a strength reduction process for computing the safety factor in terms of strength. Indeed. this advanced software has already been used to obtain new stability solutions for a wide range of practical problems including soil anchors, slopes, foundations under combined loading, excavations, tunnels, mine workings, and sinkholes.

SCIENTIFIC AND ENGINEERING APPROACHES

• Rigorous upper and lower bound elements to bracket exact collapse load from above and below, thus providing an inbuilt error estimator. High precision mixed elements to estimate collapse load accurately with minimum number of elements in the mesh.
• State of the art high-performance interior-point solver, for large and sparse conic programs, that utilises heterogeneous computing systems with massively parallel GPUs and multicore CPUs. Plus, capability to handle quadratic objective terms and semidefinite cone constraints which allow a much greater range of important problems to be tackled by the solver (such as those with complex yield criteria).
• Fully automatic adaptive mesh-refinement schemes that are particularly robust for purely frictional materials (sands). These are based on so-called optimum mesh size (and discretisation error) distribution with the incorporation of refinement/coarsening and controlled stretching of the elements.
• Efficient methods for transforming common geomaterial yield conditions into conic form. This allows highly robust solvers be employed for the resulting optimization problem, and will lead to dramatic speed-ups in the computation of limit loads.
• Possibility to conduct stochastic analysis on the basis of the random fields concept. Random distributions of a specified parameter are generated and the analysis is carried out in a Monte Carlo fashion. Rather than a single value of bearing capacity or safety factor, the end result is probability distributions of these quantities.

APPLICATIONS

• Soil anchoring systems
• Retaining walls, bridge abutments, dams and slopes
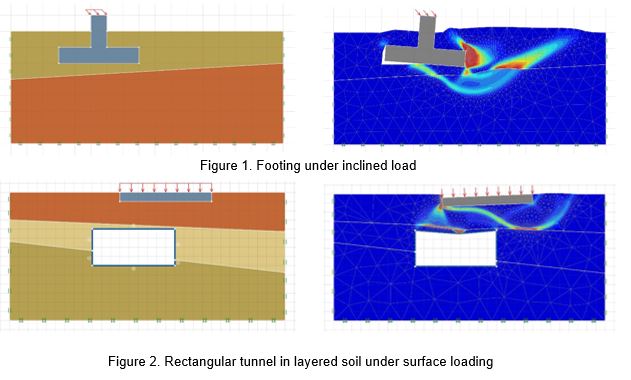
• Foundations under combined loading
• Excavations, sinkholes, mine workings and tunnels
• Piles and soil columns